Donisthorpe’s Miracle
October 1, 2018
This is part of the Wikipedia entry on Wordsworth Donisthorpe:
Wordsworth Donisthorpe (Leeds, 24 March 1847 – Shottermill, 30 January 1914) was an English individualist anarchist and inventor, pioneer of cinematography and chess enthusiast. His father was George E. Donisthorpe, also an inventor; his brother, Horace Donisthorpe, was a myrmecologist [i.e. studied ants].
In 1885 Donisthorpe was co-founder of the British Chess Association and the British Chess Club.
Donisthorpe filed for a patent in 1876, for a film camera, which he named a "kinesigraph." Although unsuccessfully at first, in 1890 he produced, together with his cousin W. C. Crofts, a moving picture of London's Trafalgar Square. In 1889 they already patented this camera, and the projector necessary to show the motion frames.
___________
So, a creative iconoclast.
Now, from Olimpiu Urcan recently:
In its chess column on page 2, the Morning Postissue of January 14, 1899 published the following letter from the ever ingenious Wordsworth Donisthorpe (1847-1914):
Sir, - I send you a problem which was constructed under unprecedented circumstances. Being somewhat of a practical joker, last Thursday at the British Chess Club, I took the first half dozen pieces that happened to be lying on a board, and placing them at haphazard in a line on the knight's file, I announced a mate with the pawn in seven moves. Some members who happened to be present, including Mr. Gunsberg, Mr. Guest, and Mr. Mortimer, set to work at the solution, in the belief that it was a bona fideproblem by Klett. You may imagine my surprise when, at the end of half an hour or so, an extremely ingenious solution was discovered in precisely seven moves, and subsequent analysis showed that the problem is sound. I venture to christen it "The Miracle." Some of your mathematical readers may perhaps like to calculate the odds against such an occurrence, which immensely exceed the probabilities against a whist player playing 13 trumps.
- Yours, &c.,
Wordsworth Donisthorpe
British Chess Club, Jan. 12
The study in question is attached herewith.
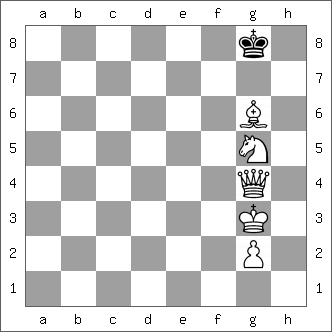
White to move and mate in 7 with the pawn
__________
Notes: I received the position by email without the solution. One cannot use an analytical engine on it because it will just find the mate in 2 with 1.Qd7 Kf8 2.Qf7#
Thus, one has to sit down and like Gunsberg, Guest and Mortimer work out the moves. I did at least an hour each night for three nights. Two thoughts were to clear out the pieces and advance the pawn or to force the king to come down to the pawn.
I finally got what I believe is the solution and I will post it as part of this thread in two days.
I think that a few chesstalkers will get the solution right off and put me to shame.
The Morning Post was a conservative daily newspaper published in London from 1772 to 1937, when it was acquired by The Daily Telegraph.
October 1, 2018
This is part of the Wikipedia entry on Wordsworth Donisthorpe:
Wordsworth Donisthorpe (Leeds, 24 March 1847 – Shottermill, 30 January 1914) was an English individualist anarchist and inventor, pioneer of cinematography and chess enthusiast. His father was George E. Donisthorpe, also an inventor; his brother, Horace Donisthorpe, was a myrmecologist [i.e. studied ants].
In 1885 Donisthorpe was co-founder of the British Chess Association and the British Chess Club.
Donisthorpe filed for a patent in 1876, for a film camera, which he named a "kinesigraph." Although unsuccessfully at first, in 1890 he produced, together with his cousin W. C. Crofts, a moving picture of London's Trafalgar Square. In 1889 they already patented this camera, and the projector necessary to show the motion frames.
___________
So, a creative iconoclast.
Now, from Olimpiu Urcan recently:
In its chess column on page 2, the Morning Postissue of January 14, 1899 published the following letter from the ever ingenious Wordsworth Donisthorpe (1847-1914):
Sir, - I send you a problem which was constructed under unprecedented circumstances. Being somewhat of a practical joker, last Thursday at the British Chess Club, I took the first half dozen pieces that happened to be lying on a board, and placing them at haphazard in a line on the knight's file, I announced a mate with the pawn in seven moves. Some members who happened to be present, including Mr. Gunsberg, Mr. Guest, and Mr. Mortimer, set to work at the solution, in the belief that it was a bona fideproblem by Klett. You may imagine my surprise when, at the end of half an hour or so, an extremely ingenious solution was discovered in precisely seven moves, and subsequent analysis showed that the problem is sound. I venture to christen it "The Miracle." Some of your mathematical readers may perhaps like to calculate the odds against such an occurrence, which immensely exceed the probabilities against a whist player playing 13 trumps.
- Yours, &c.,
Wordsworth Donisthorpe
British Chess Club, Jan. 12
The study in question is attached herewith.
White to move and mate in 7 with the pawn
__________
Notes: I received the position by email without the solution. One cannot use an analytical engine on it because it will just find the mate in 2 with 1.Qd7 Kf8 2.Qf7#
Thus, one has to sit down and like Gunsberg, Guest and Mortimer work out the moves. I did at least an hour each night for three nights. Two thoughts were to clear out the pieces and advance the pawn or to force the king to come down to the pawn.
I finally got what I believe is the solution and I will post it as part of this thread in two days.
I think that a few chesstalkers will get the solution right off and put me to shame.
The Morning Post was a conservative daily newspaper published in London from 1772 to 1937, when it was acquired by The Daily Telegraph.


Comment