Re: Heterodox chess (chess variants) thread 2.0
During my testing, a strange-looking endgame position came up and I thought I could tweak it just a bit to become the very first Chivalry, Graveyard Edition problem position. It demonstrates a few key properties of this variant.
(Btw, I like 'memorial squares' better than 'plague squares' because it fits in with the chivalry nature of the game, that is, any piece would rather be killed than dishonor those who perished in the battle).
So here's a position in which you must replace all Black pawns with trees (remember, I used pine trees from hobby railroad kits as memorial markers). There is one exception: the d7 Black Pawn is actually a Black Pawn.
Also, the White Knight is actually a White Champion, so in a proper diagram, its icon would have the shield-and-cross to indicate that. Also, I'm limited to showing an 8x8 board, but there could be 2 extra ranks on the top end of the board and 2 extra files on right side of the board and it wouldn't influence anything, not even the move notation I'll give for the solution.
So Black has one Pawn on d7, the other Black Pawns in the diagram are all trees / memorials which do not move. An interesting property of Chivalry is that King and Champion can checkmate lone King. It takes a while to do it especially on a 10x10 board, but it can be done (likewise with King and ArchBishop against lone King). It may be that with larger board, 50-move draw rule may have to be extended a bit -- maybe 60-move rule.
What this means here is that Black is likely to lose eventually even should he succeed in taking White's Pawns. But the actual optimal win sequence is very instructive for this variant.
You must remember that I made stalemate a LOSS for the side in stalemate. So this problem is White to play and mate in 4, and is this case, 'mate' means either checkmate or stalemate.
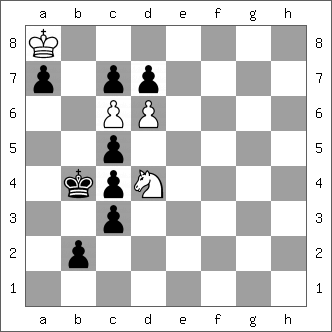
So again, all Black Pawns are trees / memorials except d7 (actual Black Pawn), meaning they do not move and cannot be passed over except by Knight and Champion. It's White to move, and there is a forced win for White... but how many moves will it take with best play from Black?
.
.
.
.
.
.
.
.
Ok, so here it is:
1. Kb7
This already illustrates something: Black's King cannot escape out from the trees via b3 and c2, NOR by a2 and b1! The White Champion covers ALL of those squares (remember, Champion moves like a Knight and a King, but in Chivalry which is the 10x10 board version, the Champion has the additional 2x3 and 3x2 L-shaped moves.
1. ... Ka4
Also possible are 1.... Ka5 and 1.... Pd7xc6, lets ignore them for the moment.
2. Pb6!
This illustrates that when a Pawn in Chivalry, Graveyard Edition is blocked by a Memorial (and only by a Memorial, not by any opposing Pawn or Piece), it is entitled to move one square to either side, even if doing so cannot lead to forward progress later on (that is, the Pawn is totally blockaded from forward progress by Memorials).
Here, the move takes away any move for Black's Pawn on d7 which leads to....
2. ... Kb4 or Ka5 or Ka3
These are the only possible moves. They all lead to the same fate, but one of them gives Black extra moves of survival.
3. Cc2
If the Black King was moved to a3 or b4, then this move is giving check. The King on a3 being moved 3....a2 means White plays 4.Ka6 for win by stalemate. The King on a3 or b4 or a5 being moved 3....Ka4 also gives 4.Ka6 for win by stalemate. And finally, the King on b4 or a5 being moved 3....Kb5 leads to 4.Cb3 for win by stalemate. All these lines have White winning by stalemate in 4 moves or less.
However, if the Black King had moved 2....Kb4 it could now be moved 3....Ka5. This offers Black more moves of life:
3....Ka5 4.Ca1! (not 4.Cb3+ 5.Kb5 and the White Champion has no good continuation)
Now Black has 3 possible King moves.
(i) 4....Kb5 5.Cb3. 1-0 by stalemate
(ii) 4....Kb4 5.Ka6 and now either
(a) 5....Ka4 6.Ca2 1-0 by stalemate
(b) 5....Ka3 6.Ka5 1-0 by stalemate
(iii) 4....Ka4 5.Ka6 and now either
(a) 5....Ka3 6.Ka5 1-0 by stalemate
(b) 5....Kb4 6.Ca2+ Ka4 7.b7 1-0 by stalemate.
And that last variation shows, barring any refutation someone may find, White requires 7 moves to win with best play.
Ok, let's look at if Black had played
1....Ka5
White still plays the sideways move
2.Pb6!
Black can only move his King, to either a4 or b4. If Black plays it to b4, then White replies with 3.Cc2 and that line has already been covered above. So now let's look at
2.... Ka4
Here White is being given the opportunity to play
3.Ka6
which leaves Black with only 2 possible replies:
(i) 3....Ka3 4.Ka5 and it is already 1-0 by stalemate because the White Champion covers both b3 and a2.
(ii) 3....Kb4 4.Cc2+ Ka4 (only move) 5.Ca1 and now either
(a) 5....Ka3 6.Ka5 and it's 1-0 by stalemate, or
(b) 5....Kb4 6.Ca2+ Ka4 7.b7 and it's 1-0 by stalemate
So substituting 1....Ka5 for 1....Ka4 leads to White requiring 7 moves again to win by stalemate. The final possibility is
Finally we have...
1.... d7xc6
This allows White to recapture with
2.Kxc6
Now if Black plays 2....Ka5, he is quickly lost:
2.... Ka5
3.Cb3+ Ka6 (only move)
4.Cb4#
An even quicker loss is
2.... Ka3
3.Kb5 and it's 1-0 by stalemate (the Champion covers the b3 and a2 squares)
So there is left for Black
2.... Ka4
and this is met with
3.Kb6 Kb4 (...Ka3 loses by stalemate to Kb5 as indicated above)
4.Cc2+ Ka4 (only move)
5.d7 and it is 1-0 by stalemate.
So it appears the final answer is that Black can hang on and make White take 7 moves to win by stalemate with best play.
Quite an amazing problem! It shows not only some new complexities involving the range and power of the Champion, but also some intricacies caused by memorial squares and the rare sideways Pawn move.
And in addition, it shows that changing the stalemate rule to a loss for the side stalemated not only avoids long, drawn-out endgames where the vastly superior side has to avoid stalemate and sometimes settle for a draw (a terrible, 'cheapo' thing to allow when one side has been outplayed imo), but shows how new and beautiful lines can be found based on forcing the losing side into loss by stalemate.
So here is the full analysis (hopefully no mistakes):
Probably no-one including Kevin will play this out... but I totally enjoyed creating it and documenting it for posterity! 
Originally posted by Kevin Pacey
View Post
During my testing, a strange-looking endgame position came up and I thought I could tweak it just a bit to become the very first Chivalry, Graveyard Edition problem position. It demonstrates a few key properties of this variant.
(Btw, I like 'memorial squares' better than 'plague squares' because it fits in with the chivalry nature of the game, that is, any piece would rather be killed than dishonor those who perished in the battle).
So here's a position in which you must replace all Black pawns with trees (remember, I used pine trees from hobby railroad kits as memorial markers). There is one exception: the d7 Black Pawn is actually a Black Pawn.
Also, the White Knight is actually a White Champion, so in a proper diagram, its icon would have the shield-and-cross to indicate that. Also, I'm limited to showing an 8x8 board, but there could be 2 extra ranks on the top end of the board and 2 extra files on right side of the board and it wouldn't influence anything, not even the move notation I'll give for the solution.
So Black has one Pawn on d7, the other Black Pawns in the diagram are all trees / memorials which do not move. An interesting property of Chivalry is that King and Champion can checkmate lone King. It takes a while to do it especially on a 10x10 board, but it can be done (likewise with King and ArchBishop against lone King). It may be that with larger board, 50-move draw rule may have to be extended a bit -- maybe 60-move rule.
What this means here is that Black is likely to lose eventually even should he succeed in taking White's Pawns. But the actual optimal win sequence is very instructive for this variant.
You must remember that I made stalemate a LOSS for the side in stalemate. So this problem is White to play and mate in 4, and is this case, 'mate' means either checkmate or stalemate.
So again, all Black Pawns are trees / memorials except d7 (actual Black Pawn), meaning they do not move and cannot be passed over except by Knight and Champion. It's White to move, and there is a forced win for White... but how many moves will it take with best play from Black?
.
.
.
.
.
.
.
.
Ok, so here it is:
1. Kb7
This already illustrates something: Black's King cannot escape out from the trees via b3 and c2, NOR by a2 and b1! The White Champion covers ALL of those squares (remember, Champion moves like a Knight and a King, but in Chivalry which is the 10x10 board version, the Champion has the additional 2x3 and 3x2 L-shaped moves.
1. ... Ka4
Also possible are 1.... Ka5 and 1.... Pd7xc6, lets ignore them for the moment.
2. Pb6!
This illustrates that when a Pawn in Chivalry, Graveyard Edition is blocked by a Memorial (and only by a Memorial, not by any opposing Pawn or Piece), it is entitled to move one square to either side, even if doing so cannot lead to forward progress later on (that is, the Pawn is totally blockaded from forward progress by Memorials).
Here, the move takes away any move for Black's Pawn on d7 which leads to....
2. ... Kb4 or Ka5 or Ka3
These are the only possible moves. They all lead to the same fate, but one of them gives Black extra moves of survival.
3. Cc2
If the Black King was moved to a3 or b4, then this move is giving check. The King on a3 being moved 3....a2 means White plays 4.Ka6 for win by stalemate. The King on a3 or b4 or a5 being moved 3....Ka4 also gives 4.Ka6 for win by stalemate. And finally, the King on b4 or a5 being moved 3....Kb5 leads to 4.Cb3 for win by stalemate. All these lines have White winning by stalemate in 4 moves or less.
However, if the Black King had moved 2....Kb4 it could now be moved 3....Ka5. This offers Black more moves of life:
3....Ka5 4.Ca1! (not 4.Cb3+ 5.Kb5 and the White Champion has no good continuation)
Now Black has 3 possible King moves.
(i) 4....Kb5 5.Cb3. 1-0 by stalemate
(ii) 4....Kb4 5.Ka6 and now either
(a) 5....Ka4 6.Ca2 1-0 by stalemate
(b) 5....Ka3 6.Ka5 1-0 by stalemate
(iii) 4....Ka4 5.Ka6 and now either
(a) 5....Ka3 6.Ka5 1-0 by stalemate
(b) 5....Kb4 6.Ca2+ Ka4 7.b7 1-0 by stalemate.
And that last variation shows, barring any refutation someone may find, White requires 7 moves to win with best play.
Ok, let's look at if Black had played
1....Ka5
White still plays the sideways move
2.Pb6!
Black can only move his King, to either a4 or b4. If Black plays it to b4, then White replies with 3.Cc2 and that line has already been covered above. So now let's look at
2.... Ka4
Here White is being given the opportunity to play
3.Ka6
which leaves Black with only 2 possible replies:
(i) 3....Ka3 4.Ka5 and it is already 1-0 by stalemate because the White Champion covers both b3 and a2.
(ii) 3....Kb4 4.Cc2+ Ka4 (only move) 5.Ca1 and now either
(a) 5....Ka3 6.Ka5 and it's 1-0 by stalemate, or
(b) 5....Kb4 6.Ca2+ Ka4 7.b7 and it's 1-0 by stalemate
So substituting 1....Ka5 for 1....Ka4 leads to White requiring 7 moves again to win by stalemate. The final possibility is
Finally we have...
1.... d7xc6
This allows White to recapture with
2.Kxc6
Now if Black plays 2....Ka5, he is quickly lost:
2.... Ka5
3.Cb3+ Ka6 (only move)
4.Cb4#
An even quicker loss is
2.... Ka3
3.Kb5 and it's 1-0 by stalemate (the Champion covers the b3 and a2 squares)
So there is left for Black
2.... Ka4
and this is met with
3.Kb6 Kb4 (...Ka3 loses by stalemate to Kb5 as indicated above)
4.Cc2+ Ka4 (only move)
5.d7 and it is 1-0 by stalemate.
So it appears the final answer is that Black can hang on and make White take 7 moves to win by stalemate with best play.
Quite an amazing problem! It shows not only some new complexities involving the range and power of the Champion, but also some intricacies caused by memorial squares and the rare sideways Pawn move.
And in addition, it shows that changing the stalemate rule to a loss for the side stalemated not only avoids long, drawn-out endgames where the vastly superior side has to avoid stalemate and sometimes settle for a draw (a terrible, 'cheapo' thing to allow when one side has been outplayed imo), but shows how new and beautiful lines can be found based on forcing the losing side into loss by stalemate.
So here is the full analysis (hopefully no mistakes):
Code:
1. Kb7 Ka4
(1....Ka5 2.Pb6! Ka4
(2....Kb4 transposes to main line)
3.Ka6 Kb4
(3....Ka3 4.Ka5 1-0 stalemate)
4.Cc2+ Ka4 (only move) 5.Ca1 Kb4
(5....Ka3 6.Ka5 1-0 stalemate)
6.Ca2+ Ka4 7.b7 1-0 by stalemate)
(1....d7xc6 2.Kxc6 Ka4
(2....Ka5 3.Cb3+ Ka6 (only move) 4.Cb4# 1-0)
(2....Ka3 3.Kb5 1-0 by stalemate)
3.Kb6 Kb4
(3...Ka3 3.Kb5 1-0 stalemate)
4.Cc2+ Ka4 (only move) 5.d7 1-0 stalemate)
2. Pb6! Kb4
(2....Ka5 3.Cc2 4.Ka4
(4.Kb5 Cb3 1-0 by stalemate)
Ka6 1-0 by stalemate)
(2....Ka3 3.Cc2+ Ka4
(3....Ka2 4.any move 1-0 stalemate)
4.Ka6 1-0 by stalemate)
3. Cc2+ Ka5
4. Ca1! Ka4
(4....Kb4 5.Ka6 Ka4
(5....Ka3 6.Ka5 1-0 stalemate)
6.Ca2 1-0 stalemate)
(4....Kb5 5.Cb3. 1-0 stalemate)
5. Ka6 Kb4
(5....Ka3 6.Ka5 1-0 stalemate)
6. Ca2+ Ka4
7. b7 1-0 by stalemate



Comment